第1776题：CONTINUE

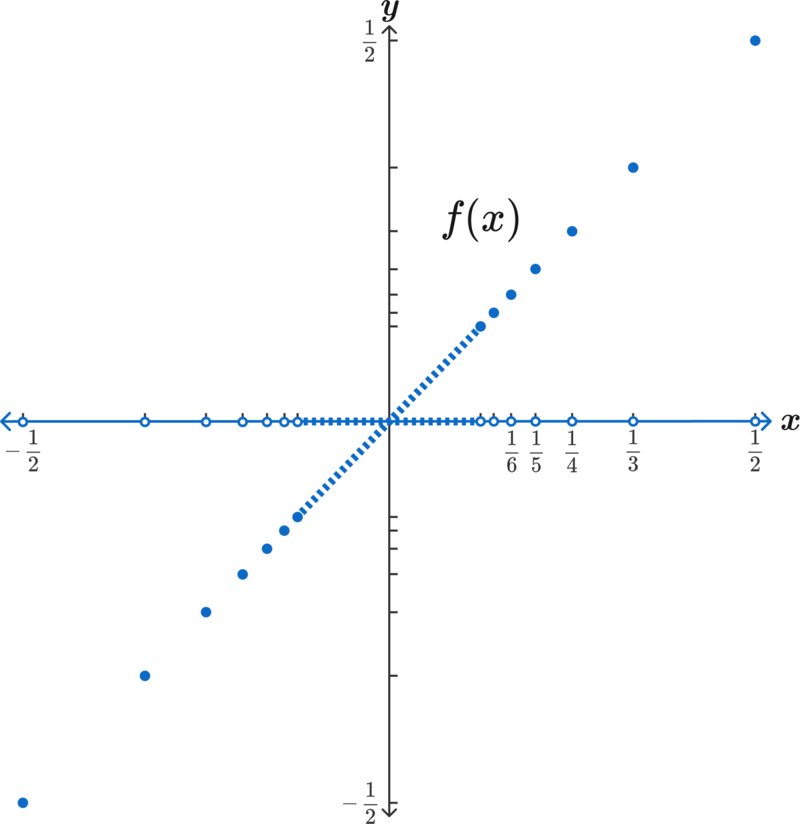
The value of $f$ is 0, except for at $\dfrac{1}{n}$ points, which lie on the line $y=x$ .

What about $\displaystyle\lim_{x \to \frac{1}{3}} f(x)\$ ?  And,  for the most interesting question, What is $\lim\limits_{x \to 0} f(x)\$ ? 

A. $\lim\limits_{x \to \frac{1}{3}} f(x)$ does not exist, $\lim\limits_{x \to 0} f(x)$ does not exist.

B. $\lim\limits_{x \to \frac{1}{3}} f(x)=\dfrac{1}{3}$ , $\lim\limits_{x \to 0} f(x)$  does not exist.

C. $\lim\limits_{x \to \frac{1}{3}} f(x)=0$ , $\lim\limits_{x \to 0} f(x)$  does not exist.

C. $\lim\limits_{x \to \frac{1}{3}} f(x)=0$ , $\lim\limits_{x \to 0} f(x)=0$ .

There is a TIP for this question.

From www.brilliant.org.