第1366题：Latus Rectum

The length of the Latus Rectum of an ellipse is equal to(  ).

A. its diameter

B. four times its focal length

C. $\dfrac{2a^2}{b}$ , where a and b are one half of the major and minor diameters, respectively

D. $\dfrac{2b^2}{a}$ , where a and b are one half of the major and minor diameters, respectively

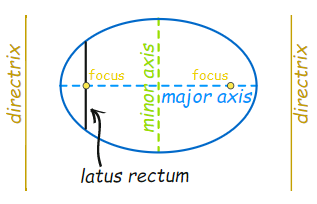
Latus Rectum

The latus rectum (no, it is not a rude word!) runs parallel to the directrix and passes through the focus. Its length:

1) In a parabola, is four times the focal length

2) In a circle, is the diameter

3) In an ellipse, is $\dfrac{2b^2}{a}$ (where a and b are one half of the major and minor diameter).